代码随想录

参与本项目，贡献其他语言版本的代码，拥抱开源，让更多学习算法的小伙伴们受益！

# 47.全排列 II

力扣题目链接 (opens new window)

给定一个可包含重复数字的序列 nums ，按任意顺序 返回所有不重复的全排列。

示例 1：

  • 输入：nums = [1,1,2]
  • 输出： [[1,1,2], [1,2,1], [2,1,1]]

示例 2：

  • 输入：nums = [1,2,3]
  • 输出：[[1,2,3],[1,3,2],[2,1,3],[2,3,1],[3,1,2],[3,2,1]]

提示：

  • 1 <= nums.length <= 8
  • -10 <= nums[i] <= 10

# 算法公开课

《代码随想录》算法视频公开课 (opens new window)：回溯算法求解全排列，如何去重？| LeetCode：47.全排列 II (opens new window)，相信结合视频再看本篇题解，更有助于大家对本题的理解。

# 思路

这道题目和46.全排列 (opens new window)的区别在与给定一个可包含重复数字的序列，要返回所有不重复的全排列。

这里又涉及到去重了。

在40.组合总和II (opens new window) 、90.子集II (opens new window)我们分别详细讲解了组合问题和子集问题如何去重。

那么排列问题其实也是一样的套路。

还要强调的是去重一定要对元素进行排序，这样我们才方便通过相邻的节点来判断是否重复使用了。

我以示例中的 [1,1,2]为例 （为了方便举例，已经排序）抽象为一棵树，去重过程如图：

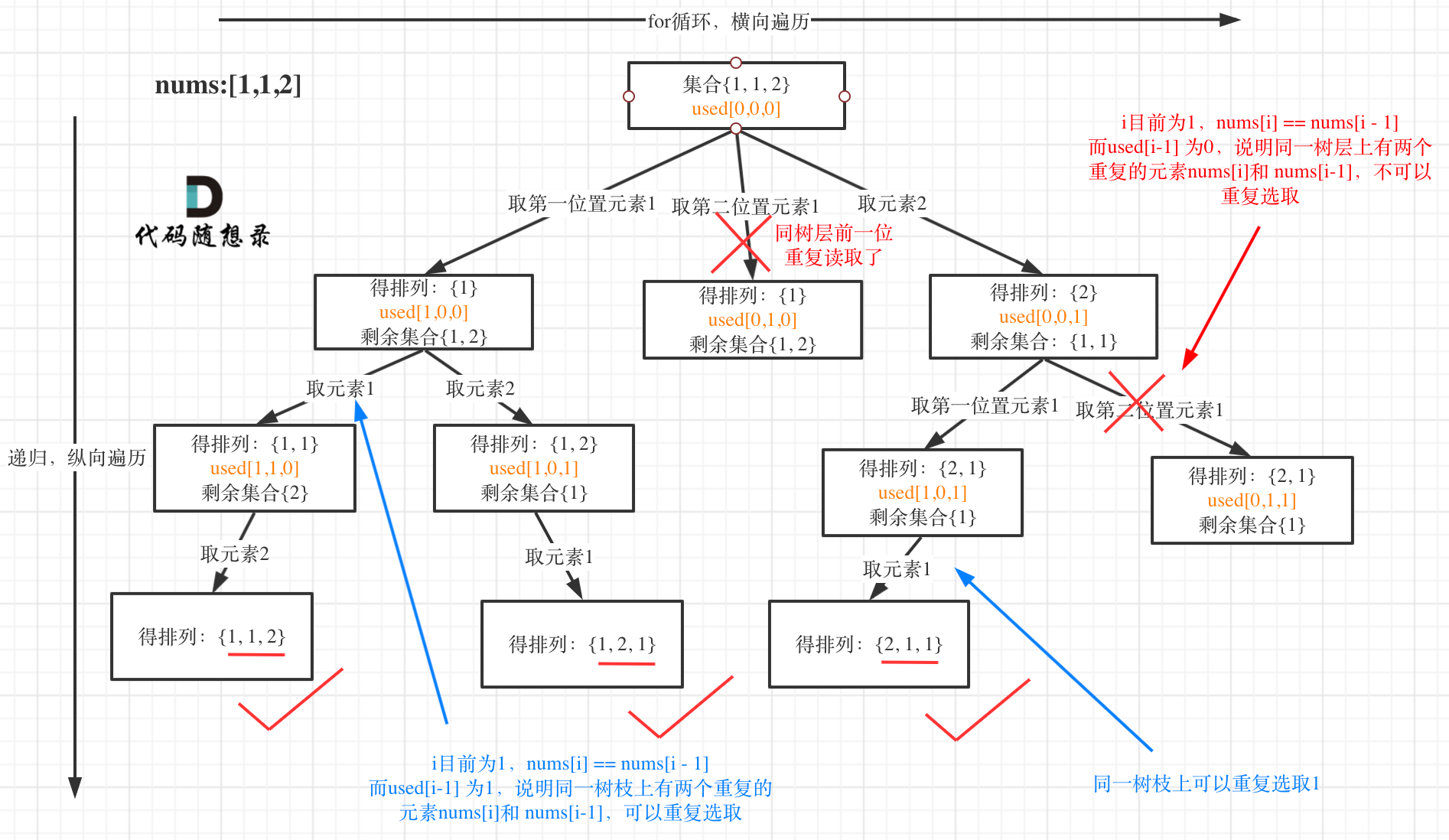
47.全排列II1

图中我们对同一树层，前一位（也就是nums[i-1]）如果使用过，那么就进行去重。

一般来说：组合问题和排列问题是在树形结构的叶子节点上收集结果，而子集问题就是取树上所有节点的结果。

在46.全排列 (opens new window)中已经详细讲解了排列问题的写法，在40.组合总和II (opens new window) 、90.子集II (opens new window)中详细讲解了去重的写法，所以这次我就不用回溯三部曲分析了，直接给出代码，如下：

class Solution {
private:
    vector<vector<int>> result;
    vector<int> path;
    void backtracking (vector<int>& nums, vector<bool>& used) {
        // 此时说明找到了一组
        if (path.size() == nums.size()) {
            result.push_back(path);
            return;
        }
        for (int i = 0; i < nums.size(); i++) {
            // used[i - 1] == true，说明同一树枝nums[i - 1]使用过
            // used[i - 1] == false，说明同一树层nums[i - 1]使用过
            // 如果同一树层nums[i - 1]使用过则直接跳过
            if (i > 0 && nums[i] == nums[i - 1] && used[i - 1] == false) {
                continue;
            }
            if (used[i] == false) {
                used[i] = true;
                path.push_back(nums[i]);
                backtracking(nums, used);
                path.pop_back();
                used[i] = false;
            }
        }
    }
public:
    vector<vector<int>> permuteUnique(vector<int>& nums) {
        result.clear();
        path.clear();
        sort(nums.begin(), nums.end()); // 排序
        vector<bool> used(nums.size(), false);
        backtracking(nums, used);
        return result;
    }
};

// 时间复杂度: 最差情况所有元素都是唯一的。复杂度和全排列1都是 O(n! * n) 对于 n 个元素一共有 n! 中排列方案。而对于每一个答案，我们需要 O(n) 去复制最终放到 result 数组
// 空间复杂度: O(n) 回溯树的深度取决于我们有多少个元素
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
  • 时间复杂度: O(n! * n)
  • 空间复杂度: O(n)

# 拓展

大家发现，去重最为关键的代码为：

if (i > 0 && nums[i] == nums[i - 1] && used[i - 1] == false) {
    continue;
}
1
2
3

如果改成 used[i - 1] == true， 也是正确的!，去重代码如下：

if (i > 0 && nums[i] == nums[i - 1] && used[i - 1] == true) {
    continue;
}
1
2
3

这是为什么呢，就是上面我刚说的，如果要对树层中前一位去重，就用used[i - 1] == false，如果要对树枝前一位去重用used[i - 1] == true。

对于排列问题，树层上去重和树枝上去重，都是可以的，但是树层上去重效率更高！

这么说是不是有点抽象？

来来来，我就用输入: [1,1,1] 来举一个例子。

树层上去重(used[i - 1] == false)，的树形结构如下：

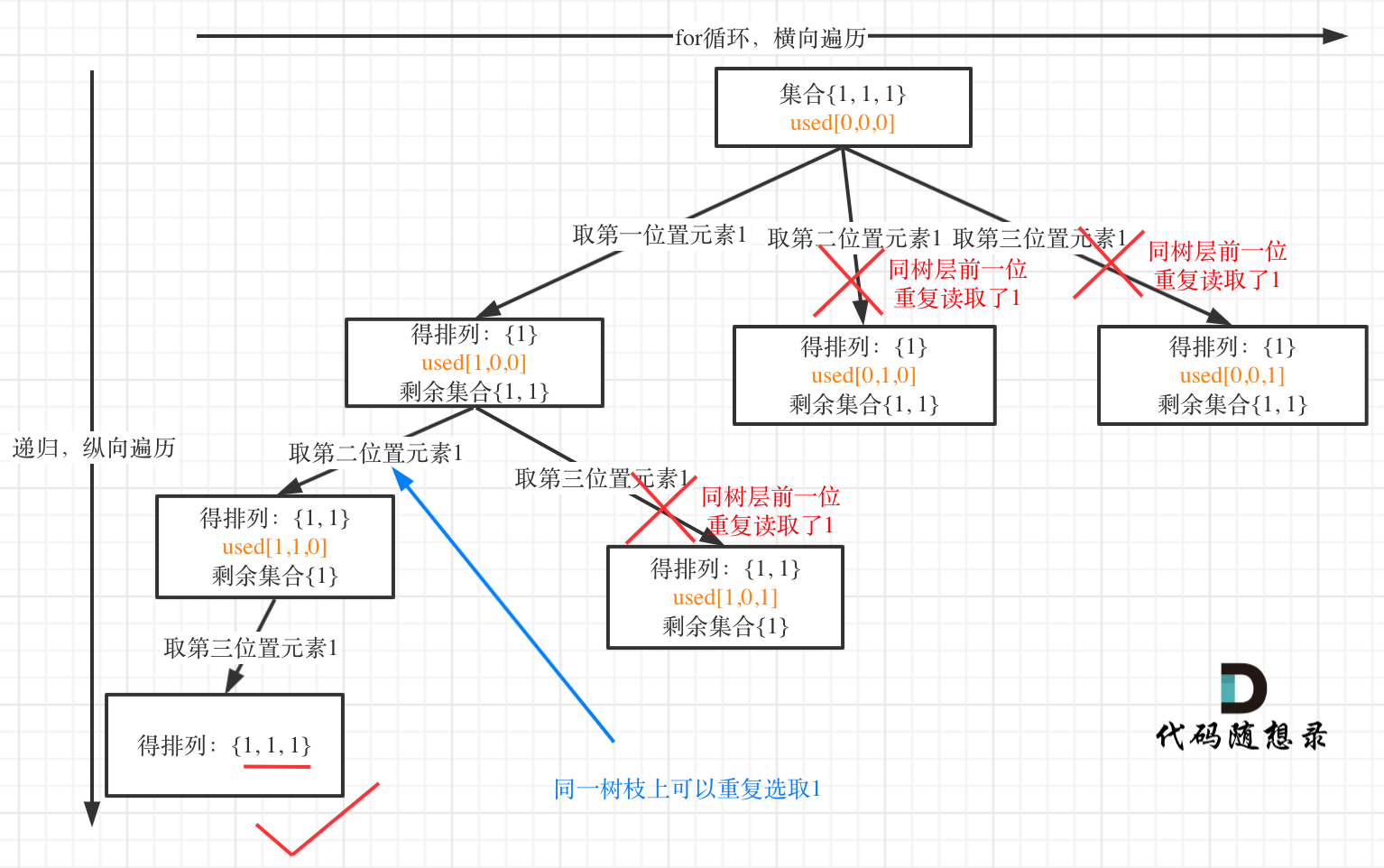
47.全排列II2

树枝上去重（used[i - 1] == true）的树型结构如下：

47.全排列II3

大家应该很清晰的看到，树层上对前一位去重非常彻底，效率很高，树枝上对前一位去重虽然最后可以得到答案，但是做了很多无用搜索。

# 总结

这道题其实还是用了我们之前讲过的去重思路，但有意思的是，去重的代码中，这么写：

if (i > 0 && nums[i] == nums[i - 1] && used[i - 1] == false) {
    continue;
}
1
2
3

和这么写：

if (i > 0 && nums[i] == nums[i - 1] && used[i - 1] == true) {
    continue;
}
1
2
3

都是可以的，这也是很多同学做这道题目困惑的地方，知道used[i - 1] == false也行而used[i - 1] == true也行，但是就想不明白为啥。

所以我通过举[1,1,1]的例子，把这两个去重的逻辑分别抽象成树形结构，大家可以一目了然：为什么两种写法都可以以及哪一种效率更高！

这里可能大家又有疑惑，既然 used[i - 1] == false也行而used[i - 1] == true也行，那为什么还要写这个条件呢？

直接这样写 不就完事了？

if (i > 0 && nums[i] == nums[i - 1]) {
    continue;
}
1
2
3

其实并不行，一定要加上 used[i - 1] == false或者used[i - 1] == true，因为 used[i - 1] 要一直是 true 或者一直是false 才可以，而不是 一会是true 一会又是false。 所以这个条件要写上。

是不是豁然开朗了！！

# 其他语言版本

# Java

class Solution {
    //存放结果
    List<List<Integer>> result = new ArrayList<>();
    //暂存结果
    List<Integer> path = new ArrayList<>();

    public List<List<Integer>> permuteUnique(int[] nums) {
        boolean[] used = new boolean[nums.length];
        Arrays.fill(used, false);
        Arrays.sort(nums);
        backTrack(nums, used);
        return result;
    }

    private void backTrack(int[] nums, boolean[] used) {
        if (path.size() == nums.length) {
            result.add(new ArrayList<>(path));
            return;
        }
        for (int i = 0; i < nums.length; i++) {
            // used[i - 1] == true，说明同⼀树⽀nums[i - 1]使⽤过
            // used[i - 1] == false，说明同⼀树层nums[i - 1]使⽤过
            // 如果同⼀树层nums[i - 1]使⽤过则直接跳过
            if (i > 0 && nums[i] == nums[i - 1] && used[i - 1] == false) {
                continue;
            }
            //如果同⼀树⽀nums[i]没使⽤过开始处理
            if (used[i] == false) {
                used[i] = true;//标记同⼀树⽀nums[i]使⽤过，防止同一树枝重复使用
                path.add(nums[i]);
                backTrack(nums, used);
                path.remove(path.size() - 1);//回溯，说明同⼀树层nums[i]使⽤过，防止下一树层重复
                used[i] = false;//回溯
            }
        }
    }
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37

Python

class Solution:
    def permuteUnique(self, nums):
        nums.sort()  # 排序
        result = []
        self.backtracking(nums, [], [False] * len(nums), result)
        return result

    def backtracking(self, nums, path, used, result):
        if len(path) == len(nums):
            result.append(path[:])
            return
        for i in range(len(nums)):
            if (i > 0 and nums[i] == nums[i - 1] and not used[i - 1]) or used[i]:
                continue
            used[i] = True
            path.append(nums[i])
            self.backtracking(nums, path, used, result)
            path.pop()
            used[i] = False
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20

# Go

var (
    res [][]int
    path  []int
    st    []bool   // state的缩写
)
func permuteUnique(nums []int) [][]int {
    res, path = make([][]int, 0), make([]int, 0, len(nums))
    st = make([]bool, len(nums))
    sort.Ints(nums)
    dfs(nums, 0)
    return res
}

func dfs(nums []int, cur int) {
    if cur == len(nums) {
        tmp := make([]int, len(path))
        copy(tmp, path)
        res = append(res, tmp)
    }
    for i := 0; i < len(nums); i++ {
        if i != 0 && nums[i] == nums[i-1] && !st[i-1] {  // 去重，用st来判别是深度还是广度
            continue
        }
        if !st[i] {
            path = append(path, nums[i])
            st[i] = true
            dfs(nums, cur + 1)
            st[i] = false
            path = path[:len(path)-1]
        }
    }
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32

# Javascript

var permuteUnique = function (nums) {
    nums.sort((a, b) => {
        return a - b
    })
    let result = []
    let path = []

    function backtracing( used) {
        if (path.length === nums.length) {
            result.push([...path])
            return
        }
        for (let i = 0; i < nums.length; i++) {
            if (i > 0 && nums[i] === nums[i - 1] && !used[i - 1]) {
                continue
            }
            if (!used[i]) {
                used[i] = true
                path.push(nums[i])
                backtracing(used)
                path.pop()
                used[i] = false
            }


        }
    }
    backtracing([])
    return result
};
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31

# TypeScript

function permuteUnique(nums: number[]): number[][] {
    nums.sort((a, b) => a - b);
    const resArr: number[][] = [];
    const usedArr: boolean[] = new Array(nums.length).fill(false);
    backTracking(nums, []);
    return resArr;
    function backTracking(nums: number[], route: number[]): void {
        if (route.length === nums.length) {
            resArr.push([...route]);
            return;
        }
        for (let i = 0, length = nums.length; i < length; i++) {
            if (i > 0 && nums[i] === nums[i - 1] && usedArr[i - 1] === false) continue;
            if (usedArr[i] === false) {
                route.push(nums[i]);
                usedArr[i] = true;
                backTracking(nums, route);
                usedArr[i] = false;
                route.pop();
            }
        }
    }
};
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23

# Swift

func permuteUnique(_ nums: [Int]) -> [[Int]] {
    let nums = nums.sorted() // 先排序，以方便相邻元素去重
    var result = [[Int]]()
    var path = [Int]()
    var used = [Bool](repeating: false, count: nums.count)
    func backtracking() {
        if path.count == nums.count {
            result.append(path)
            return
        }

        for i in 0 ..< nums.count {
            // !used[i - 1]表示同一树层nums[i - 1]使用过，直接跳过，这一步很关键！
            if i > 0, nums[i] == nums[i - 1], !used[i - 1] { continue }
            if used[i] { continue }
            used[i] = true
            path.append(nums[i])
            backtracking()
            // 回溯
            path.removeLast()
            used[i] = false
        }
    }
    backtracking()
    return result
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26

# Rust

impl Solution {
    fn backtracking(result: &mut Vec<Vec<i32>>, path: &mut Vec<i32>, nums: &Vec<i32>, used: &mut Vec<bool>) {
        let len = nums.len();
        if path.len() == len {
            result.push(path.clone());
            return;
        }
        for i in 0..len {
            if i > 0 && nums[i] == nums[i - 1] && used[i - 1] == false { continue; }
            if used[i] == false {
                used[i] = true;
                path.push(nums[i]);
                Self::backtracking(result, path, nums, used);
                path.pop();
                used[i] = false;
            }
        }
    }

    pub fn permute_unique(nums: Vec<i32>) -> Vec<Vec<i32>> {
        let mut result: Vec<Vec<i32>> = Vec::new();
        let mut path: Vec<i32> = Vec::new();
        let mut used = vec![false; nums.len()];
        let mut nums= nums;
        nums.sort();
        Self::backtracking(&mut result, &mut path, &nums, &mut used);
        result
    }
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29

# C

//临时数组
int *path;
//返回数组
int **ans;
int *used;
int pathTop, ansTop;

//拷贝path到ans中
void copyPath() {
    int *tempPath = (int*)malloc(sizeof(int) * pathTop);
    int i;
    for(i = 0; i < pathTop; ++i) {
        tempPath[i] = path[i];
    }
    ans[ansTop++] = tempPath;
}

void backTracking(int* used, int *nums, int numsSize) {
    //若path中元素个数等于numsSize，将path拷贝入ans数组中
    if(pathTop == numsSize)
        copyPath();
    int i;
    for(i = 0; i < numsSize; i++) {
        //若当前元素已被使用
        //或前一位元素与当前元素值相同但并未被使用
        //则跳过此分支
        if(used[i] || (i != 0 && nums[i] == nums[i-1] && used[i-1] == 0))
            continue;

        //将当前元素的使用情况设为True
        used[i] = 1;
        path[pathTop++] = nums[i];
        backTracking(used, nums, numsSize);
        used[i] = 0;
        --pathTop;
    }
}

int cmp(void* elem1, void* elem2) {
    return *((int*)elem1) - *((int*)elem2);
}

int** permuteUnique(int* nums, int numsSize, int* returnSize, int** returnColumnSizes){
    //排序数组
    qsort(nums, numsSize, sizeof(int), cmp);
    //初始化辅助变量
    pathTop = ansTop = 0;
    path = (int*)malloc(sizeof(int) * numsSize);
    ans = (int**)malloc(sizeof(int*) * 1000);
    //初始化used辅助数组
    used = (int*)malloc(sizeof(int) * numsSize);
    int i;
    for(i = 0; i < numsSize; i++) {
        used[i] = 0;
    }

    backTracking(used, nums, numsSize);

    //设置返回的数组的长度
    *returnSize = ansTop;
    *returnColumnSizes = (int*)malloc(sizeof(int) * ansTop);
    int z;
    for(z = 0; z < ansTop; z++) {
        (*returnColumnSizes)[z] = numsSize;
    }
    return ans;
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67

# Scala

object Solution {
  import scala.collection.mutable
  def permuteUnique(nums: Array[Int]): List[List[Int]] = {
    var result = mutable.ListBuffer[List[Int]]()
    var path = mutable.ListBuffer[Int]()
    var num = nums.sorted // 首先对数据进行排序

    def backtracking(used: Array[Boolean]): Unit = {
      if (path.size == num.size) {
        // 如果path的size等于num了，那么可以添加到结果集
        result.append(path.toList)
        return
      }
      // 循环守卫，当前元素没被使用过就进入循环体
      for (i <- num.indices if used(i) == false) {
        // 当前索引为0，不存在和前一个数字相等可以进入回溯
        // 当前索引值和上一个索引不相等，可以回溯
        // 前一个索引对应的值没有被选，可以回溯
        // 因为Scala没有continue，只能将逻辑反过来写
        if (i == 0 || (i > 0 && num(i) != num(i - 1)) || used(i-1) == false) {
          used(i) = true
          path.append(num(i))
          backtracking(used)
          path.remove(path.size - 1)
          used(i) = false
        }
      }
    }

    backtracking(new Array[Boolean](nums.length))
    result.toList
  }
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33

# C#

public class Solution
{
    public List<IList<int>> res = new List<IList<int>>();
    public List<int> path = new List<int>();
    public IList<IList<int>> PermuteUnique(int[] nums)
    {
        Array.Sort(nums);
        BackTracking(nums, new bool[nums.Length]);
        return res;
    }
    public void BackTracking(int[] nums, bool[] used)
    {
        if (nums.Length == path.Count)
        {
            res.Add(new List<int>(path));
            return;
        }
        for (int i = 0; i < nums.Length; i++)
        {
            if (i > 0 && nums[i] == nums[i - 1] && used[i - 1] == false) continue;
            if (used[i]) continue;
            path.Add(nums[i]);
            used[i] = true;
            BackTracking(nums, used);
            path.RemoveAt(path.Count - 1);
            used[i] = false;
        }
    }
}
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
上次更新：: 8/22/2024, 9:07:28 PM

← 17. 回溯周末总结 →

@2021-2024 代码随想录 版权所有 粤ICP备19156078号