- 做项目(多个C++、Java、Go、测开、前端项目) (opens new window)
- 刷算法(两个月高强度学算法) (opens new window)
- 背八股(40天挑战高频面试题) (opens new window)
本来是打算将二叉树和二叉搜索树的公共祖先问题一起讲,后来发现篇幅过长了,只能先说一说二叉树的公共祖先问题。
# 236. 二叉树的最近公共祖先
给定一个二叉树, 找到该树中两个指定节点的最近公共祖先。
百度百科中最近公共祖先的定义为:“对于有根树 T 的两个结点 p、q,最近公共祖先表示为一个结点 x,满足 x 是 p、q 的祖先且 x 的深度尽可能大(一个节点也可以是它自己的祖先)。”
例如,给定如下二叉树: root = [3,5,1,6,2,0,8,null,null,7,4]
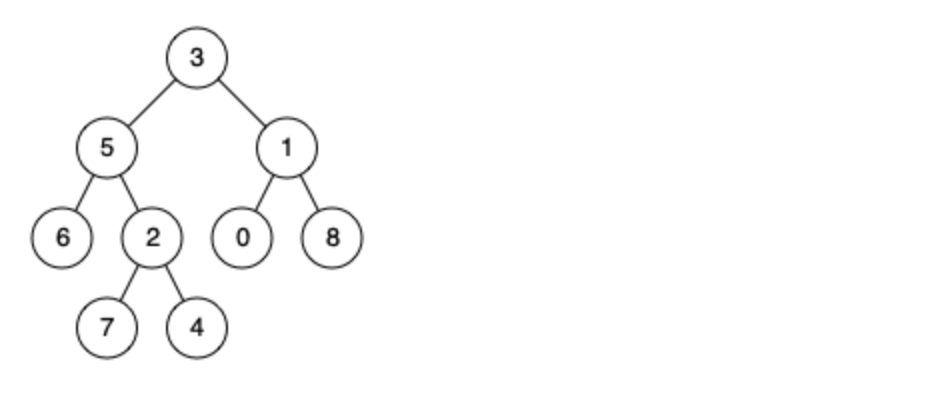
示例 1: 输入: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 1 输出: 3 解释: 节点 5 和节点 1 的最近公共祖先是节点 3。
示例 2: 输入: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 4 输出: 5 解释: 节点 5 和节点 4 的最近公共祖先是节点 5。因为根据定义最近公共祖先节点可以为节点本身。
说明:
- 所有节点的值都是唯一的。
- p、q 为不同节点且均存在于给定的二叉树中。
# 算法公开课
《代码随想录》算法视频公开课 (opens new window):自底向上查找,有点难度! | LeetCode:236. 二叉树的最近公共祖先 (opens new window),相信结合视频再看本篇题解,更有助于大家对本题的理解。
# 思路
遇到这个题目首先想的是要是能自底向上查找就好了,这样就可以找到公共祖先了。
那么二叉树如何可以自底向上查找呢?
回溯啊,二叉树回溯的过程就是从底到上。
后序遍历(左右中)就是天然的回溯过程,可以根据左右子树的返回值,来处理中节点的逻辑。
接下来就看如何判断一个节点是节点q和节点p的公共祖先呢。
首先最容易想到的一个情况:如果找到一个节点,发现左子树出现结点p,右子树出现节点q,或者 左子树出现结点q,右子树出现节点p,那么该节点就是节点p和q的最近公共祖先。 即情况一:
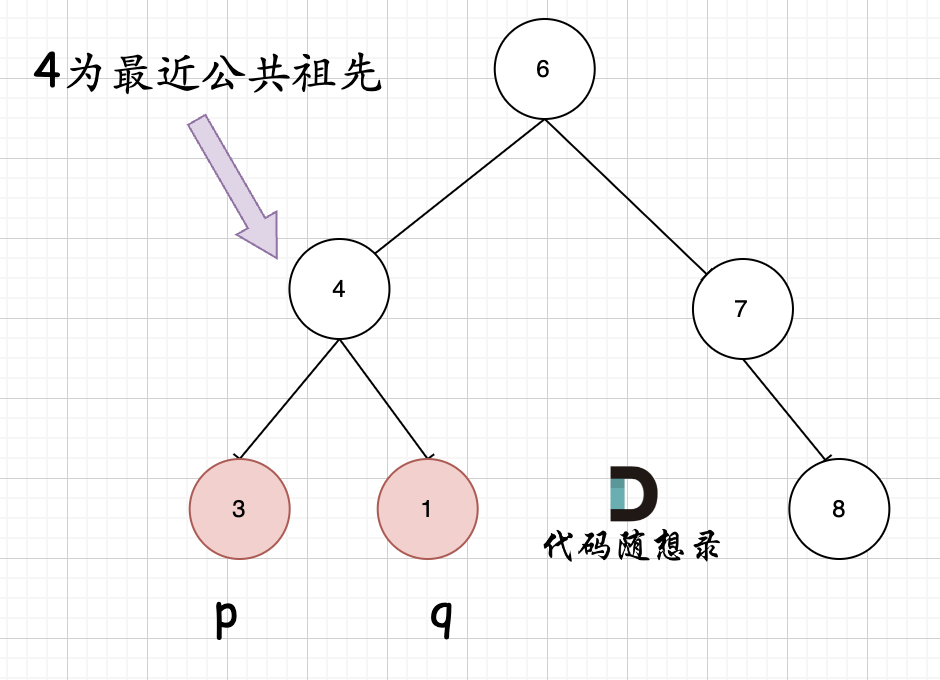
判断逻辑是 如果递归遍历遇到q,就将q返回,遇到p 就将p返回,那么如果 左右子树的返回值都不为空,说明此时的中节点,一定是q 和p 的最近祖先。
那么有录友可能疑惑,会不会左子树 遇到q 返回,右子树也遇到q返回,这样并没有找到 q 和p的最近祖先。
这么想的录友,要审题了,题目强调:二叉树节点数值是不重复的,而且一定存在 q 和 p。
但是很多人容易忽略一个情况,就是节点本身p(q),它拥有一个子孙节点q(p)。 情况二:
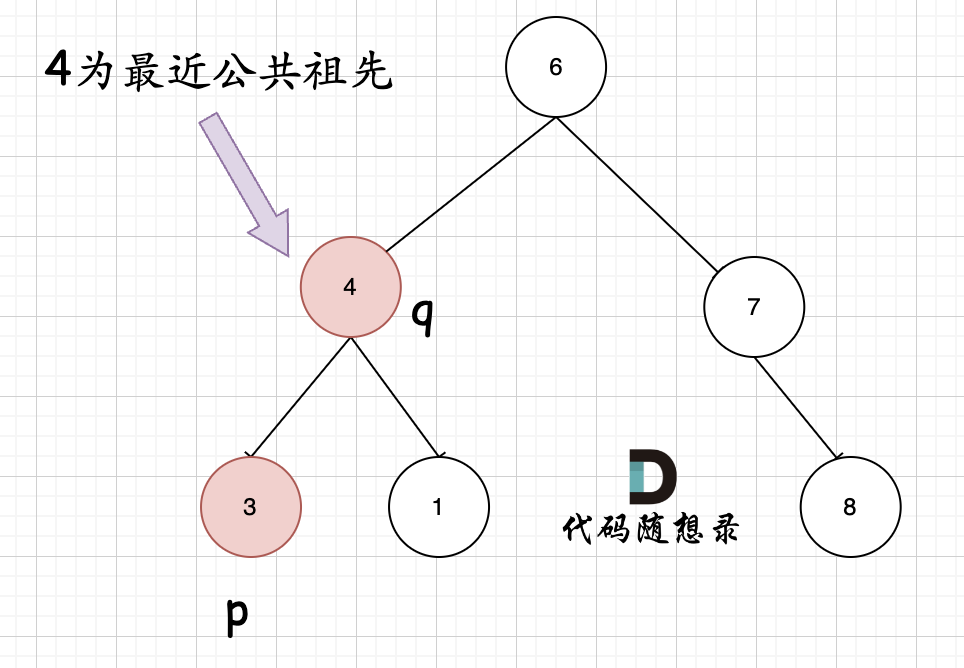
其实情况一 和 情况二 代码实现过程都是一样的,也可以说,实现情况一的逻辑,顺便包含了情况二。
因为遇到 q 或者 p 就返回,这样也包含了 q 或者 p 本身就是 公共祖先的情况。
这一点是很多录友容易忽略的,在下面的代码讲解中,可以再去体会。
递归三部曲:
- 确定递归函数返回值以及参数
需要递归函数返回值,来告诉我们是否找到节点q或者p,那么返回值为bool类型就可以了。
但我们还要返回最近公共节点,可以利用上题目中返回值是TreeNode * ,那么如果遇到p或者q,就把q或者p返回,返回值不为空,就说明找到了q或者p。
代码如下:
TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q)
- 确定终止条件
遇到空的话,因为树都是空了,所以返回空。
那么我们来说一说,如果 root == q,或者 root == p,说明找到 q p ,则将其返回,这个返回值,后面在中节点的处理过程中会用到,那么中节点的处理逻辑,下面讲解。
代码如下:
if (root == q || root == p || root == NULL) return root;
- 确定单层递归逻辑
值得注意的是 本题函数有返回值,是因为回溯的过程需要递归函数的返回值做判断,但本题我们依然要遍历树的所有节点。
我们在二叉树:递归函数究竟什么时候需要返回值,什么时候不要返回值? (opens new window)中说了 递归函数有返回值就是要遍历某一条边,但有返回值也要看如何处理返回值!
如果递归函数有返回值,如何区分要搜索一条边,还是搜索整个树呢?
搜索一条边的写法:
if (递归函数(root->left)) return ;
if (递归函数(root->right)) return ;
2
3
搜索整个树写法:
left = 递归函数(root->left); // 左
right = 递归函数(root->right); // 右
left与right的逻辑处理; // 中
2
3
看出区别了没?
在递归函数有返回值的情况下:如果要搜索一条边,递归函数返回值不为空的时候,立刻返回,如果搜索整个树,直接用一个变量left、right接住返回值,这个left、right后序还有逻辑处理的需要,也就是后序遍历中处理中间节点的逻辑(也是回溯)。
那么为什么要遍历整棵树呢?直观上来看,找到最近公共祖先,直接一路返回就可以了。
如图:
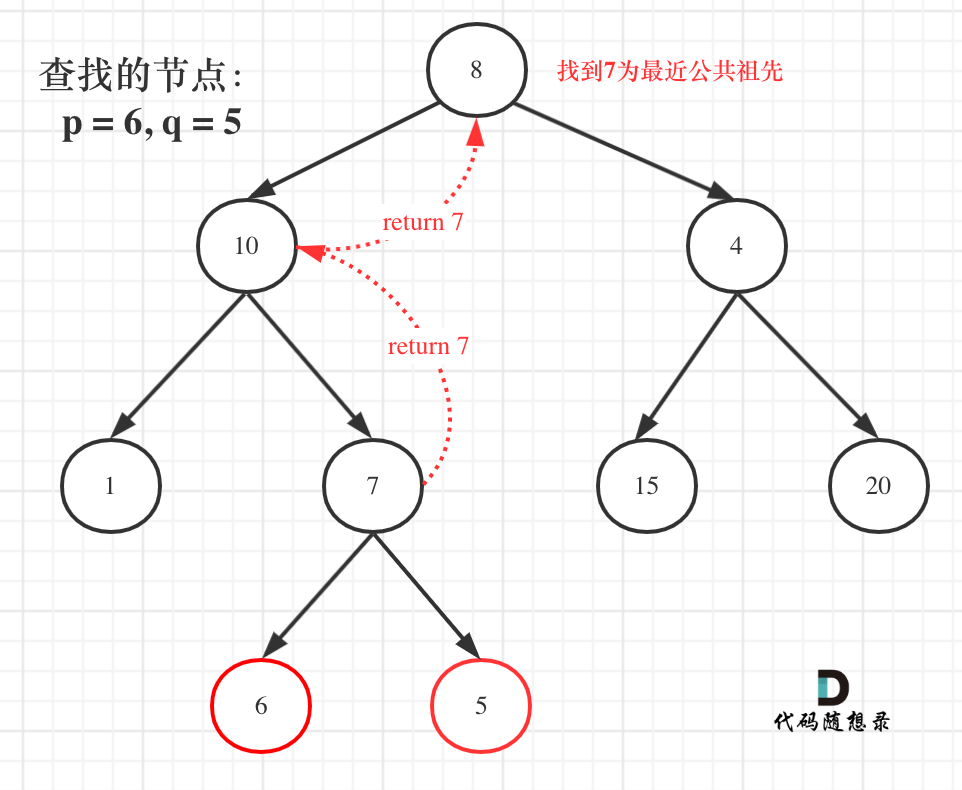
就像图中一样直接返回7。
但事实上还要遍历根节点右子树(即使此时已经找到了目标节点了),也就是图中的节点4、15、20。
因为在如下代码的后序遍历中,如果想利用left和right做逻辑处理, 不能立刻返回,而是要等left与right逻辑处理完之后才能返回。
left = 递归函数(root->left); // 左
right = 递归函数(root->right); // 右
left与right的逻辑处理; // 中
2
3
所以此时大家要知道我们要遍历整棵树。知道这一点,对本题就有一定深度的理解了。
那么先用left和right接住左子树和右子树的返回值,代码如下:
TreeNode* left = lowestCommonAncestor(root->left, p, q);
TreeNode* right = lowestCommonAncestor(root->right, p, q);
2
3
如果left 和 right都不为空,说明此时root就是最近公共节点。这个比较好理解
如果left为空,right不为空,就返回right,说明目标节点是通过right返回的,反之依然。
这里有的同学就理解不了了,为什么left为空,right不为空,目标节点通过right返回呢?
如图:

图中节点10的左子树返回null,右子树返回目标值7,那么此时节点10的处理逻辑就是把右子树的返回值(最近公共祖先7)返回上去!
这里也很重要,可能刷过这道题目的同学,都不清楚结果究竟是如何从底层一层一层传到头结点的。
那么如果left和right都为空,则返回left或者right都是可以的,也就是返回空。
代码如下:
if (left == NULL && right != NULL) return right;
else if (left != NULL && right == NULL) return left;
else { // (left == NULL && right == NULL)
return NULL;
}
2
3
4
5
6
那么寻找最小公共祖先,完整流程图如下:
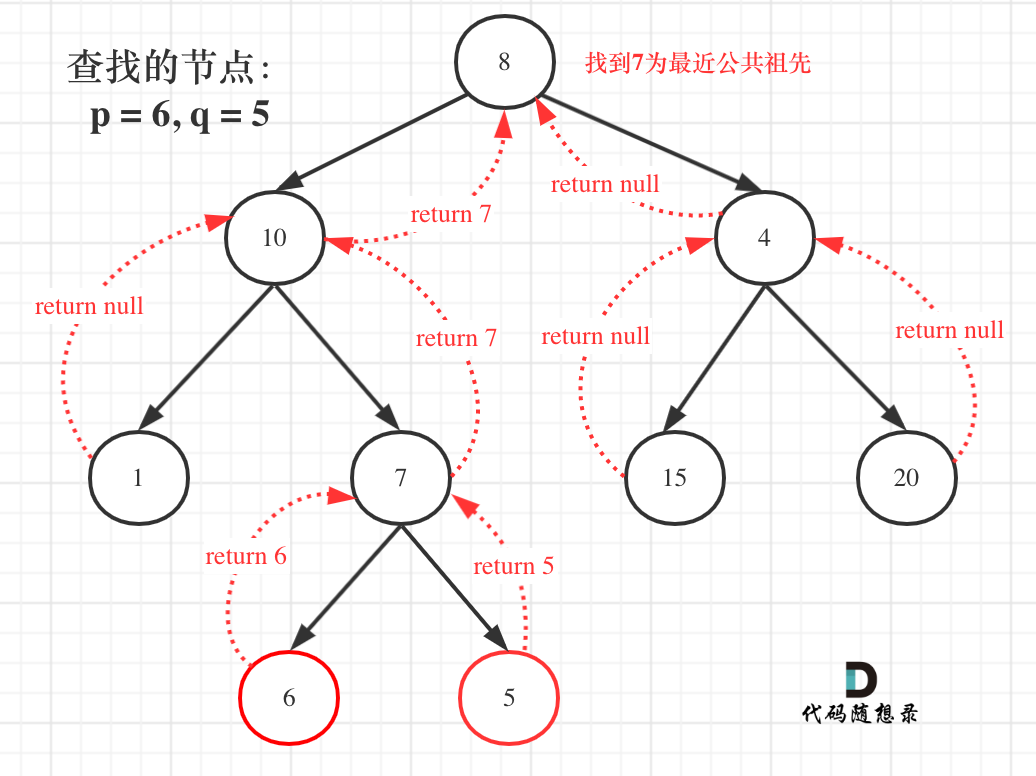
从图中,大家可以看到,我们是如何回溯遍历整棵二叉树,将结果返回给头结点的!
整体代码如下:
class Solution {
public:
TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {
if (root == q || root == p || root == NULL) return root;
TreeNode* left = lowestCommonAncestor(root->left, p, q);
TreeNode* right = lowestCommonAncestor(root->right, p, q);
if (left != NULL && right != NULL) return root;
if (left == NULL && right != NULL) return right;
else if (left != NULL && right == NULL) return left;
else { // (left == NULL && right == NULL)
return NULL;
}
}
};
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
稍加精简,代码如下:
class Solution {
public:
TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {
if (root == q || root == p || root == NULL) return root;
TreeNode* left = lowestCommonAncestor(root->left, p, q);
TreeNode* right = lowestCommonAncestor(root->right, p, q);
if (left != NULL && right != NULL) return root;
if (left == NULL) return right;
return left;
}
};
2
3
4
5
6
7
8
9
10
11
# 总结
这道题目刷过的同学未必真正了解这里面回溯的过程,以及结果是如何一层一层传上去的。
那么我给大家归纳如下三点:
求最小公共祖先,需要从底向上遍历,那么二叉树,只能通过后序遍历(即:回溯)实现从底向上的遍历方式。
在回溯的过程中,必然要遍历整棵二叉树,即使已经找到结果了,依然要把其他节点遍历完,因为要使用递归函数的返回值(也就是代码中的left和right)做逻辑判断。
要理解如果返回值left为空,right不为空为什么要返回right,为什么可以用返回right传给上一层结果。
可以说这里每一步,都是有难度的,都需要对二叉树,递归和回溯有一定的理解。
本题没有给出迭代法,因为迭代法不适合模拟回溯的过程。理解递归的解法就够了。
# 其他语言版本
# Java
递归
class Solution {
public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
if (root == null || root == p || root == q) { // 递归结束条件
return root;
}
// 后序遍历
TreeNode left = lowestCommonAncestor(root.left, p, q);
TreeNode right = lowestCommonAncestor(root.right, p, q);
if(left == null && right == null) { // 若未找到节点 p 或 q
return null;
}else if(left == null && right != null) { // 若找到一个节点
return right;
}else if(left != null && right == null) { // 若找到一个节点
return left;
}else { // 若找到两个节点
return root;
}
}
}
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
迭代
class Solution {
public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
int max = Integer.MAX_VALUE;
Stack<TreeNode> st = new Stack<>();
TreeNode cur = root, pre = null;
while (cur != null || !st.isEmpty()) {
while (cur != null) {
st.push(cur);
cur = cur.left;
}
cur = st.pop();
if (cur.right == null || cur.right == pre) {
// p/q是 中/左 或者 中/右 , 返回中
if (cur == p || cur == q) {
if ((cur.left != null && cur.left.val == max) || (cur.right != null && cur.right.val == max)) {
return cur;
}
cur.val = max;
}
// p/q是 左/右 , 返回中
if (cur.left != null && cur.left.val == max && cur.right != null && cur.right.val == max) {
return cur;
}
// MAX_VALUE 往上传递
if ((cur.left != null && cur.left.val == max) || (cur.right != null && cur.right.val == max)) {
cur.val = max;
}
pre = cur;
cur = null;
} else {
st.push(cur);
cur = cur.right;
}
}
return null;
}
}
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
# Python
递归法(版本一)
class Solution:
def lowestCommonAncestor(self, root, p, q):
if root == q or root == p or root is None:
return root
left = self.lowestCommonAncestor(root.left, p, q)
right = self.lowestCommonAncestor(root.right, p, q)
if left is not None and right is not None:
return root
if left is None and right is not None:
return right
elif left is not None and right is None:
return left
else:
return None
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
递归法(版本二)精简
class Solution:
def lowestCommonAncestor(self, root, p, q):
if root == q or root == p or root is None:
return root
left = self.lowestCommonAncestor(root.left, p, q)
right = self.lowestCommonAncestor(root.right, p, q)
if left is not None and right is not None:
return root
if left is None:
return right
return left
2
3
4
5
6
7
8
9
10
11
12
13
14
15
# Go
func lowestCommonAncestor(root, p, q *TreeNode) *TreeNode {
// check
if root == nil {
return root
}
// 相等 直接返回root节点即可
if root == p || root == q {
return root
}
// Divide
left := lowestCommonAncestor(root.Left, p, q)
right := lowestCommonAncestor(root.Right, p, q)
// Conquer
// 左右两边都不为空,则根节点为祖先
if left != nil && right != nil {
return root
}
if left != nil {
return left
}
if right != nil {
return right
}
return nil
}
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
# JavaScript
var lowestCommonAncestor = function(root, p, q) {
// 使用递归的方法
// 需要从下到上,所以使用后序遍历
// 1. 确定递归的函数
const travelTree = function(root,p,q) {
// 2. 确定递归终止条件
if(root === null || root === p || root === q) {
return root;
}
// 3. 确定递归单层逻辑
let left = travelTree(root.left,p,q);
let right = travelTree(root.right,p,q);
if(left !== null && right !== null) {
return root;
}
if(left === null) {
return right;
}
return left;
}
return travelTree(root,p,q);
};
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
# TypeScript
function lowestCommonAncestor(root: TreeNode | null, p: TreeNode | null, q: TreeNode | null): TreeNode | null {
if (root === null || root === p || root === q) return root;
const left = lowestCommonAncestor(root.left, p, q);
const right = lowestCommonAncestor(root.right, p, q);
if (left !== null && right !== null) return root;
if (left !== null) return left;
if (right !== null) return right;
return null;
};
2
3
4
5
6
7
8
9
# Scala
object Solution {
def lowestCommonAncestor(root: TreeNode, p: TreeNode, q: TreeNode): TreeNode = {
// 递归结束条件
if (root == null || root == p || root == q) {
return root
}
var left = lowestCommonAncestor(root.left, p, q)
var right = lowestCommonAncestor(root.right, p, q)
if (left != null && right != null) return root
if (left == null) return right
left
}
}
2
3
4
5
6
7
8
9
10
11
12
13
14
15
# Rust
impl Solution {
pub fn lowest_common_ancestor(
root: Option<Rc<RefCell<TreeNode>>>,
p: Option<Rc<RefCell<TreeNode>>>,
q: Option<Rc<RefCell<TreeNode>>>,
) -> Option<Rc<RefCell<TreeNode>>> {
if root.is_none() {
return root;
}
if Rc::ptr_eq(root.as_ref().unwrap(), p.as_ref().unwrap())
|| Rc::ptr_eq(root.as_ref().unwrap(), q.as_ref().unwrap()) {
return root;
}
let left = Self::lowest_common_ancestor(
root.as_ref().unwrap().borrow().left.clone(),
p.clone(),
q.clone(),
);
let right =
Self::lowest_common_ancestor(root.as_ref().unwrap().borrow().right.clone(), p, q);
match (&left, &right) {
(None, Some(_)) => right,
(Some(_), Some(_)) => root,
_ => left,
}
}
}
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
# C#
public TreeNode LowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q)
{
if (root == null || root == p || root == q) return root;
TreeNode left = LowestCommonAncestor(root.left, p, q);
TreeNode right = LowestCommonAncestor(root.right, p, q);
if (left != null && right != null) return root;
if (left == null && right != null) return right;
if (left != null && right == null) return left;
return null;
}
2
3
4
5
6
7
8
9
10
